nerolimo.blogg.se

(10) ∠BAD ≅ ∠BCA //definition of congruent angles (9) m∠BCA=m∠BAD //(2), (8), transitive property of equality (7) m∠BCA=½m∠BOA //Inscribed angle theorem (5) m∠ABO = m∠BAO //(4), base angle theorem (4) OA=OB //All radii of a circle are equal (3) m∠BAO = 90-α //(1), Angle addition postulate (1) m∠DAO = 90° //Given, AD is tangent to circle O, the tangent is perpendicular to the radius

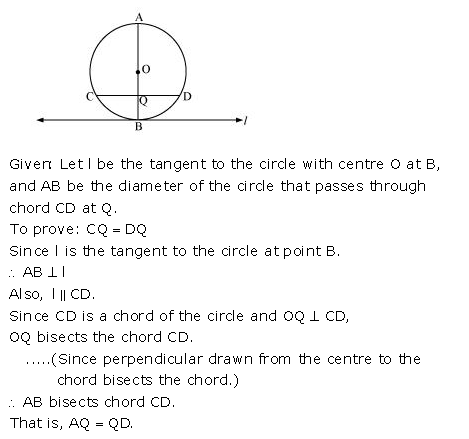
Now, ∠BCA is an inscribed angle that subtends the same arc as the central angle ∠BOA, so by the inscribed angle theorem, it is equal to half of m∠BOA, or α, and ∠BAD ≅ ∠BCA. From the sum of angles in a triangle, m∠BOA=180-2 From the base angle theorem, m∠ABO is also 90-α.

We'll draw another radius, from O to B:Īnd since all radii are equal, OA=OB and we have an isosceles triangle △AOB. Let's call ∠BAD "α", and then m∠BAO will be 90-α. Let's draw that radius, AO, so m∠DAO is 90°. StrategyĪs we're dealing with a tangent line, we'll use the fact that the tangent is perpendicular to the radius at the point it touches the circle. The Tangent-Chord Theorem states that the angle formed between a chord and a tangent line to a circle is equal to the inscribed angle on the other side of the chord: ∠BAD ≅ ∠BCA.